A) .171
B) .525
C) .571
D) .120
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Given the contingency table shown here,if a randomly chosen student attends school in an inner city location,what is the probability that it is a public school? 
A) .189
B) .333
C) .500
D) .567
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Given the contingency table shown here,find the probability that a randomly chosen employee is a line worker who plans to retire at age 65. Survey question: Do you plan on retiring or keep working when you turn 65? 
A) .227
B) .419
C) .750
D) .315
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Given the contingency table shown here,find P(A ∩ M) . 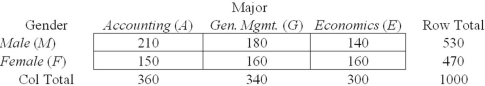
A) .210
B) .360
C) .396
D) .583
Correct Answer

verified
Correct Answer
verified
True/False
If P(A)= .20 then the odds against event A's occurrence are 4 to 1.
Correct Answer

verified
True
Correct Answer
verified
True/False
The sum of the probabilities of two mutually exclusive events is one.
Correct Answer

verified
Correct Answer
verified
True/False
The empirical view of probability is based on relative frequencies.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Given the contingency table shown here,find the joint probability that a call sampled at random out of this population is local and 2-5 minutes long. 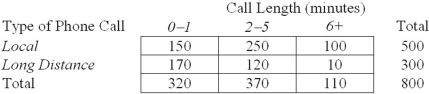
A) .5000
B) .3125
C) .4000
D) .4625
Correct Answer

verified
Correct Answer
verified
True/False
Probability is the measure of the relative likelihood that an event will occur.
Correct Answer

verified
Correct Answer
verified
True/False
Independent events are mutually exclusive.
Correct Answer

verified
False
Correct Answer
verified
Multiple Choice
The probability that event A occurs,given that event B has occurred,is an example of:
A) a marginal probability.
B) a conditional probability.
C) a joint probability.
D) more than one of the above.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Regarding the rules of probability,which of the following statements is correct?
A) If A and B are independent events,then P(B) = P(A) P(B) .
B) The sum of two mutually exclusive events is one.
C) The probability of A and its complement will sum to one.
D) If event A occurs,then its complement will also occur.
Correct Answer

verified
Correct Answer
verified
True/False
The general law of addition for probabilities says P(A or B)= P(A)+ P(B)- P(A ∩ B)
Correct Answer

verified
Correct Answer
verified
True/False
Baye's Theorem shows how to revise a prior probability to obtain a conditional or posterior probability when another event's occurrence is known.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Given the contingency table shown here,find P(V) . 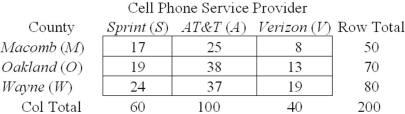
A) .20
B) .40
C) .50
D) .80
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Given the contingency table shown here,find P(B) . 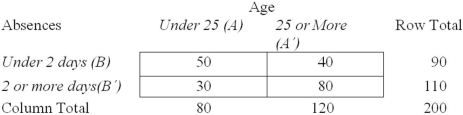
A) .85
B) .25
C) .45
D) .22
Correct Answer

verified
Correct Answer
verified
Multiple Choice
At Joe's Restaurant,80 percent of the diners are new customers (N) ,while 20 percent are returning customers (R) .Fifty percent of the new customers pay by credit card,compared with 70 percent of the regular customers.If a customer pays by credit card,what is the probability that the customer is a new customer?
A) .7407
B) .8000
C) .5400
D) .5000
Correct Answer

verified
Correct Answer
verified
Multiple Choice
From the following tree,find the probability that a randomly chosen person will not get a vaccination and will not get the flu. 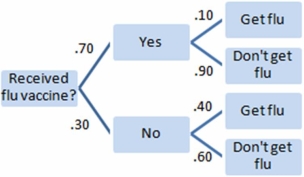
A) .18
B) .60
C) .19
D) .70
Correct Answer

verified
Correct Answer
verified
True/False
The odds of an event can be calculated by dividing the event's probability by the probability of its complement.
Correct Answer

verified
True
Correct Answer
verified
Multiple Choice
Given the contingency table shown here,find P(E) . 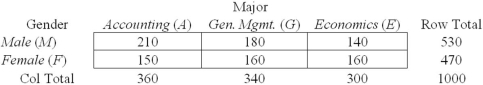
A) .180
B) .300
C) .529
D) .641
Correct Answer

verified
Correct Answer
verified
Showing 1 - 20 of 121
Related Exams